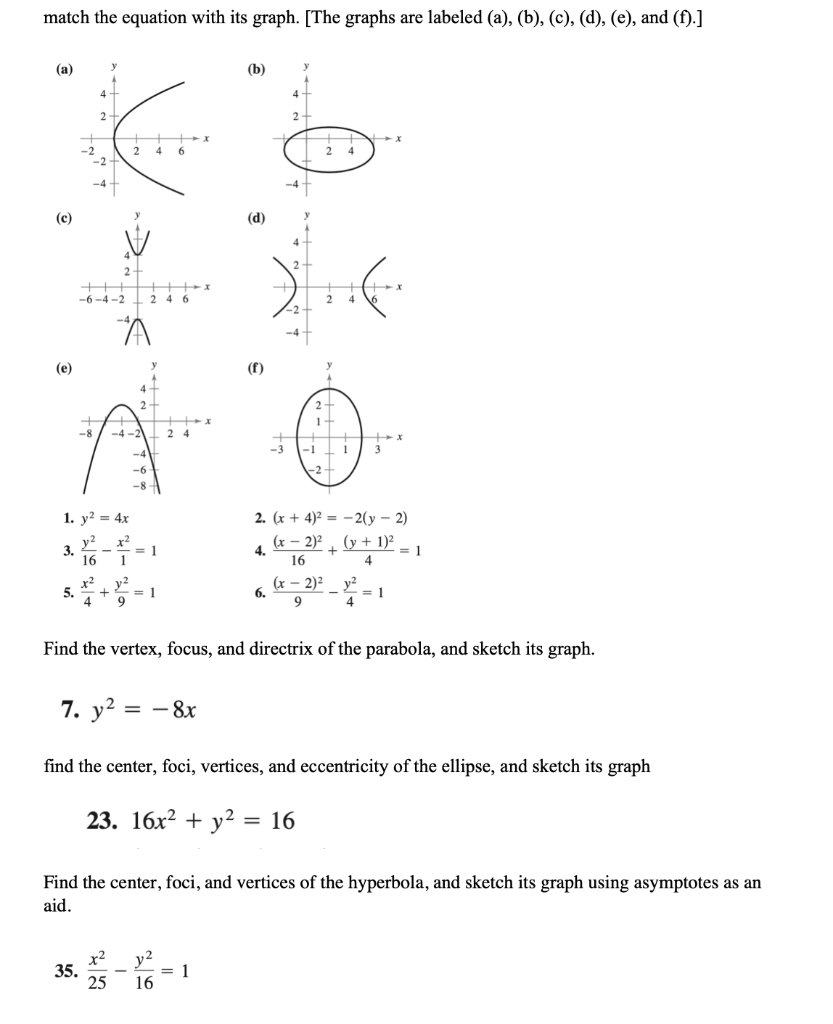
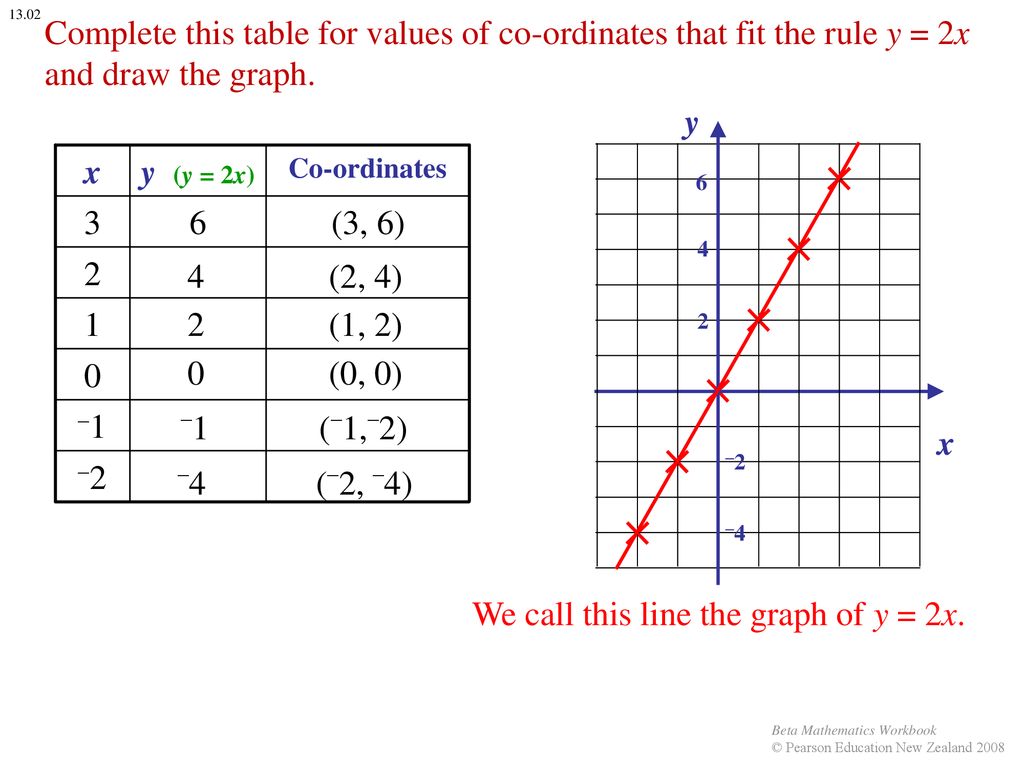
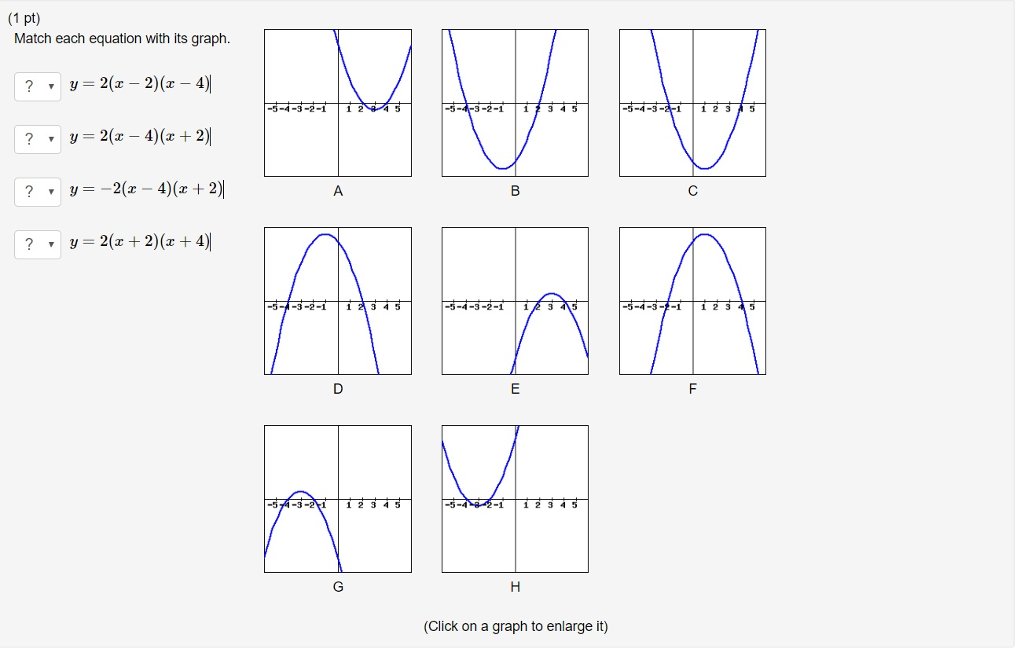
Solved Match The Equation With Its Graph The Graphs Are Chegg Com
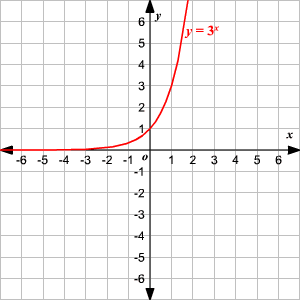
🚩 reality check #1 your function is a parabola #2 y=52/3x linear, if you mean 5 (2/3)x y=5x^27 obviously nonlinear y=15x025 y=3 y=10/x nonlinear #3 y=3/4x7/8 > a straight line if you mean (3/4)x 7/8 a curve if you mean 3/ (4x) 7/8 👍 👎 ℹ️ 🚩 Reiny The answers for the quiz are 1A 2A 3C 4BThe graph of y=(2)^x is then What you don't see in the picture is that the graph has a large number of "holes" The only points on the graph have first coordinate x=p/q where p and q are integers with no common factors other than 1 and q is odd
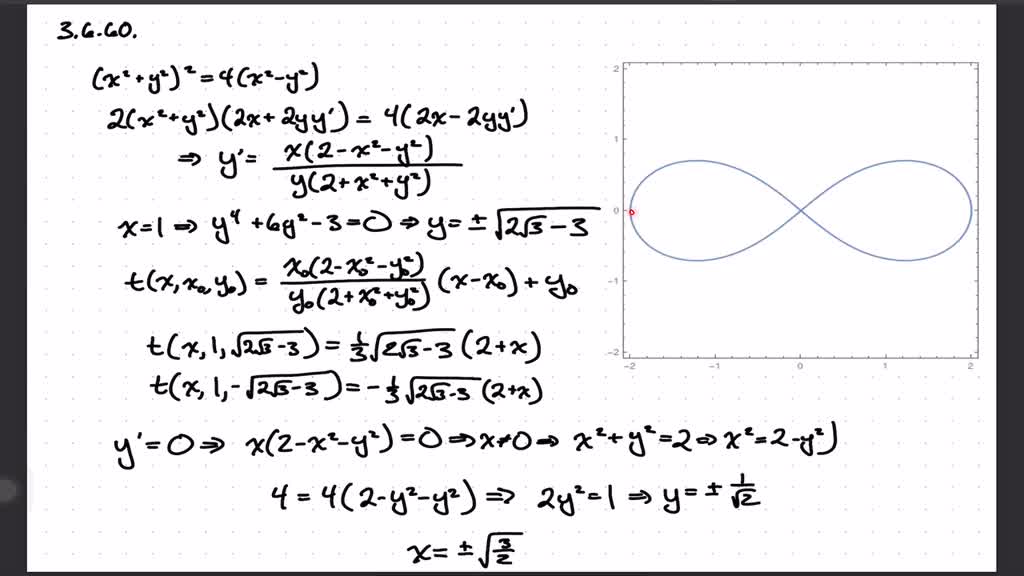
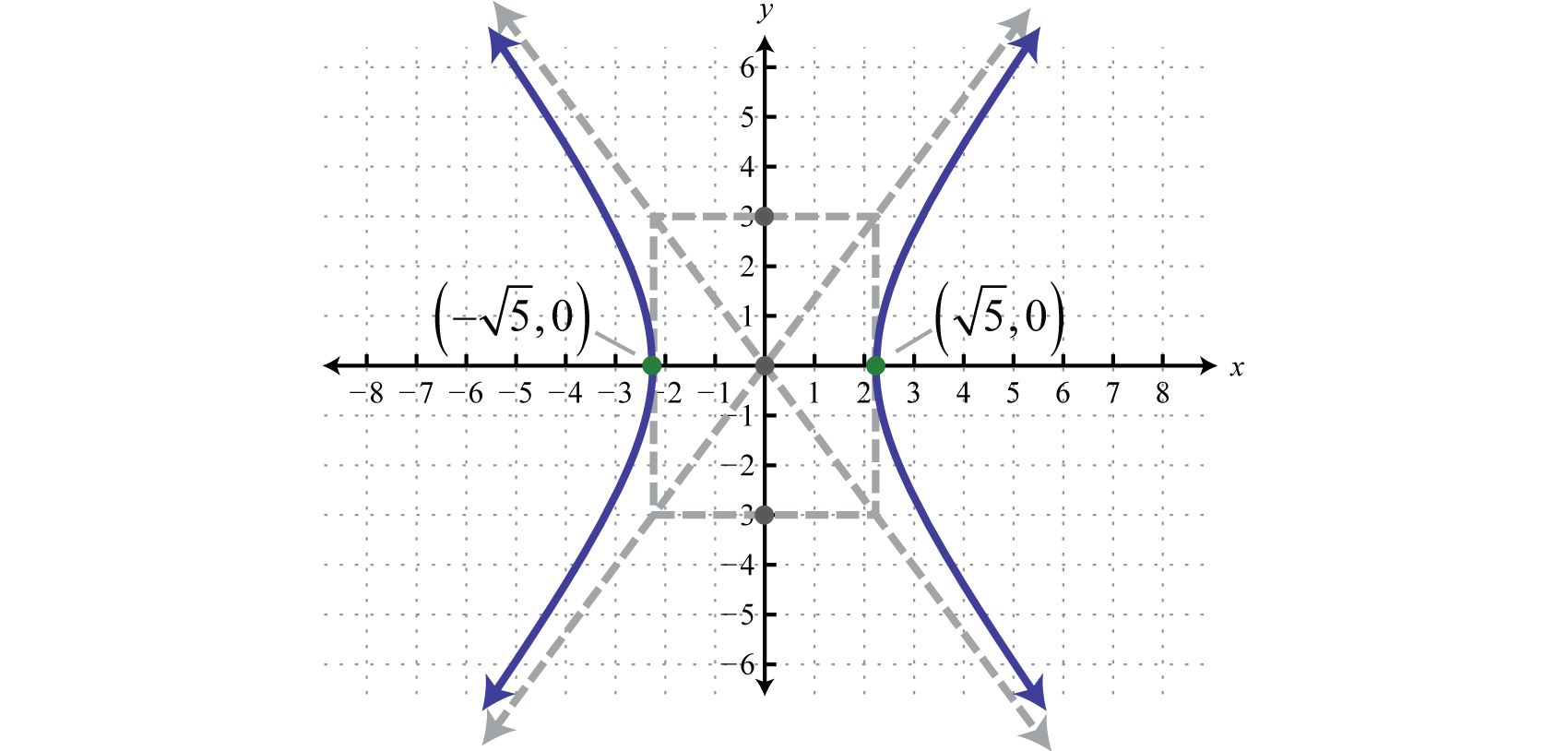
Graph y^2/4-x^2/9=1
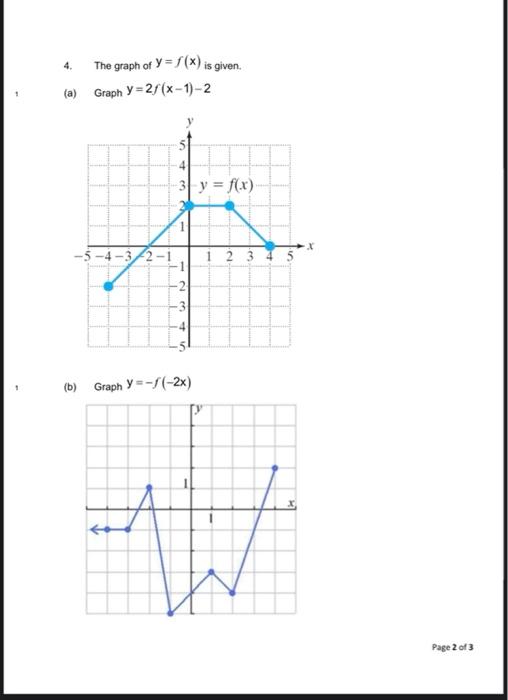
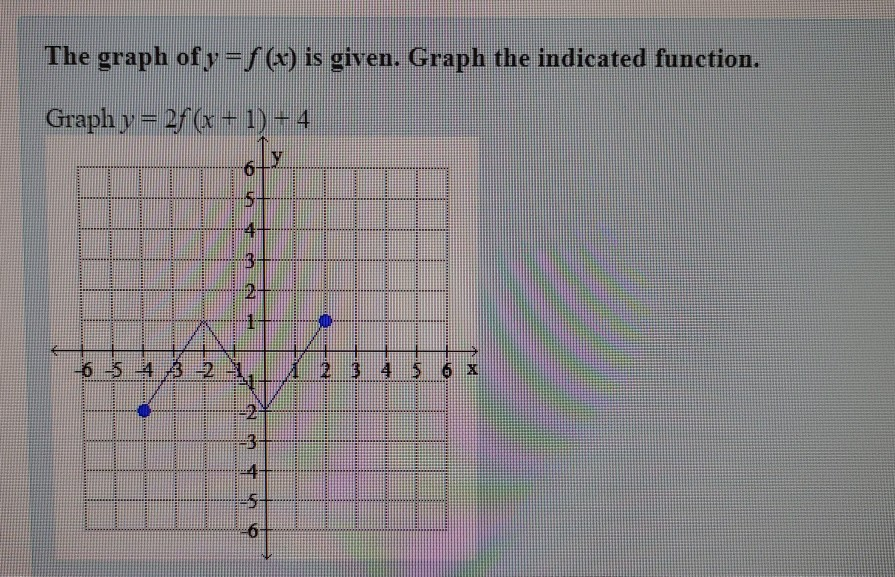
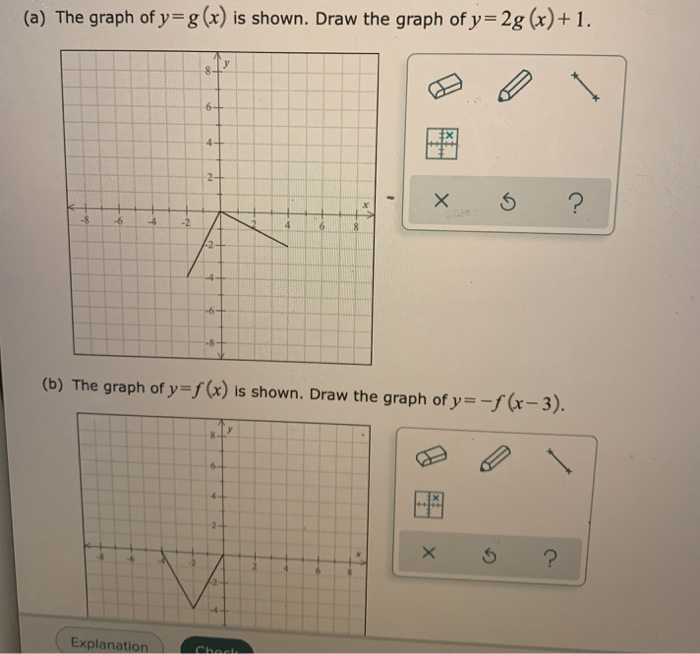
Graph y^2/4-x^2/9=1- If we think about the equation ( y 1) 2 = x for a while, we can see that x will be positive for all values of y (since any value squared will be positive) except y = −1 (at which point x = 0) In the equation ( y 1) 2 = x, the "plus 1" in brackets has the effect of moving our rotated parabola down one unit Example 8 ( y − 3) 2 = x6 6 2 T (b) The graph of y=h (x) is shown Draw the graph of
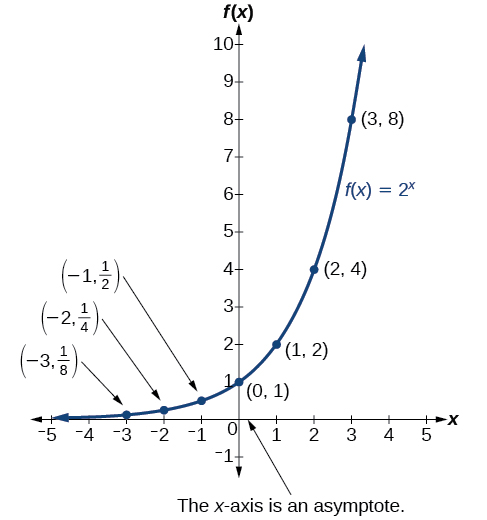
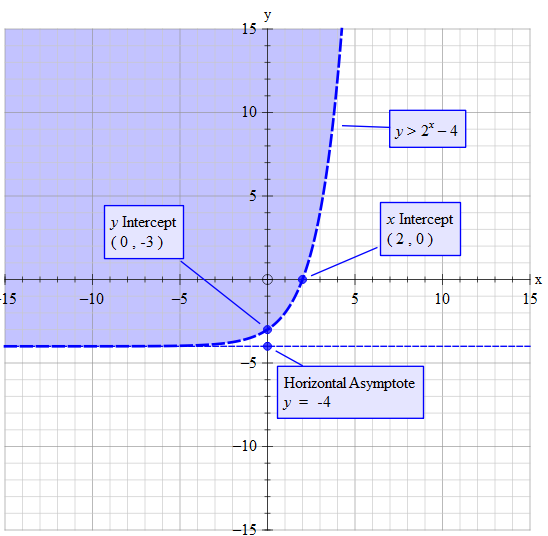
Graphs Of Exponential Functions Algebra And Trigonometry
Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition Draw the graph of y = 2x^2 – 3x – 5 and hence solve 2x^2 – 4x – 6 = 0 y = 2x^2 – 3x – 5 asked in Algebra by Darshee ( 494k points) algebraIf x2 y2 xy = 1, Then minimum and maximum value of x3y xy3 4, where x,y ∈ R https//mathstackexchangecom/q/ You already showed that f (x,y) = xy(x2 y2)4 = xy(1− xy)4, which is a quadratic function in xy It remains to find the exact range for xy For which you showed that xy ≥ −1, equality
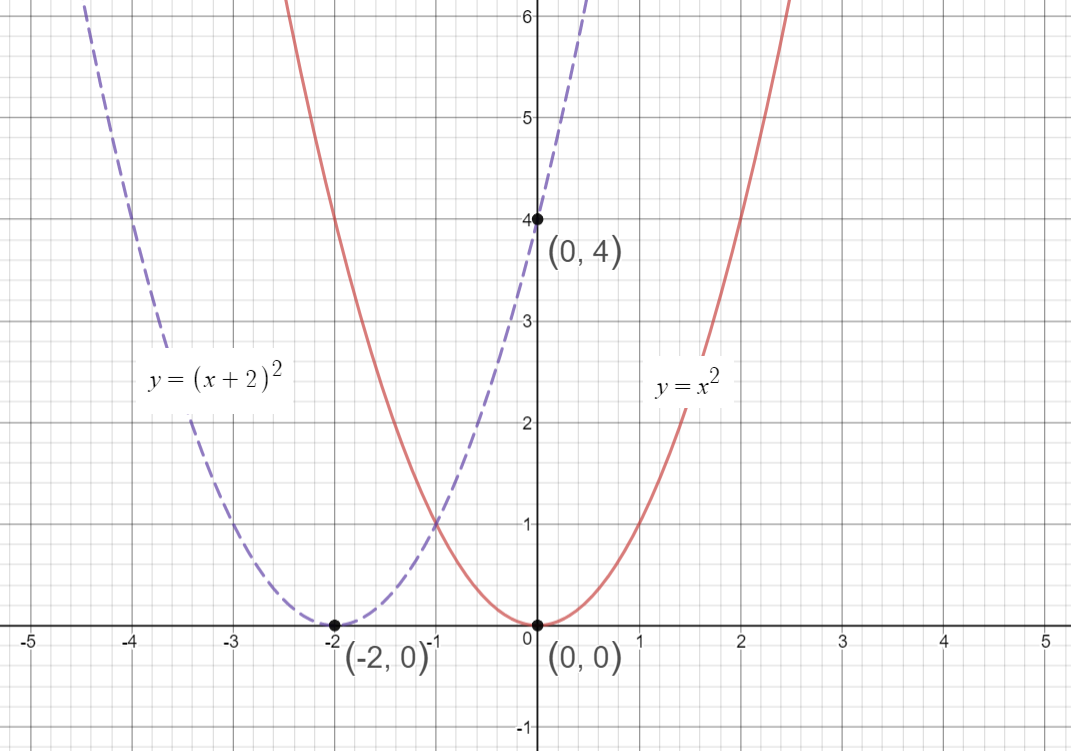
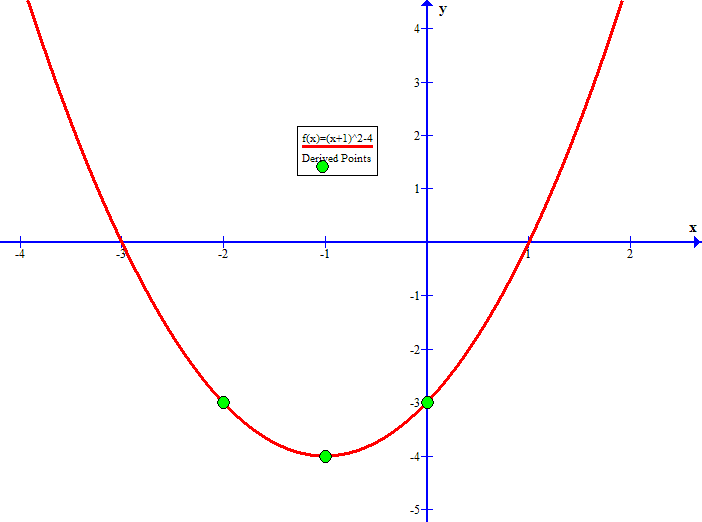
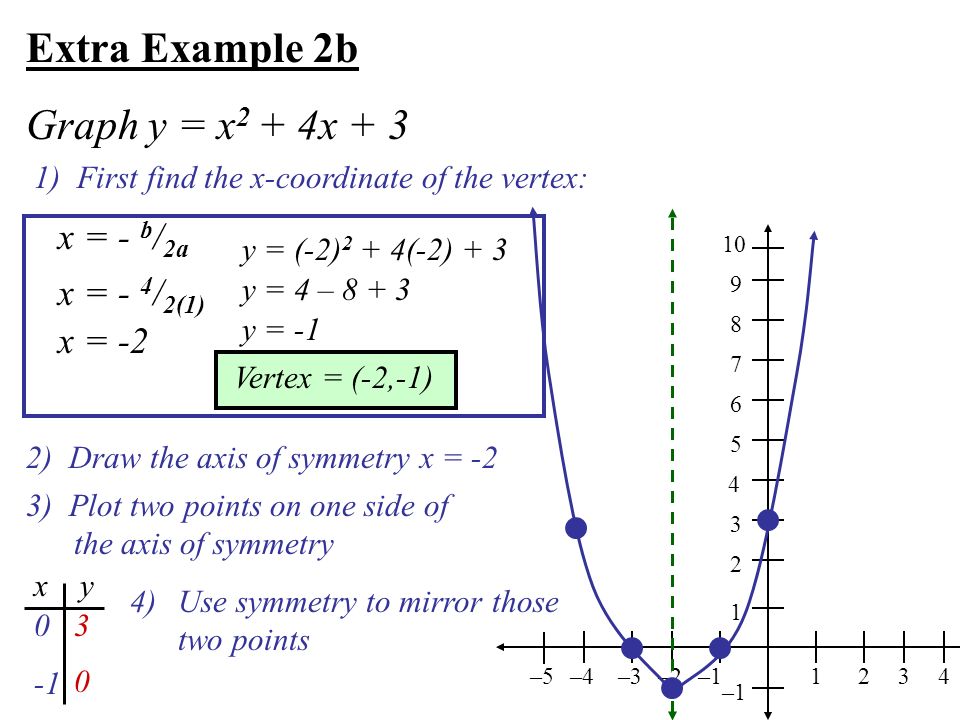
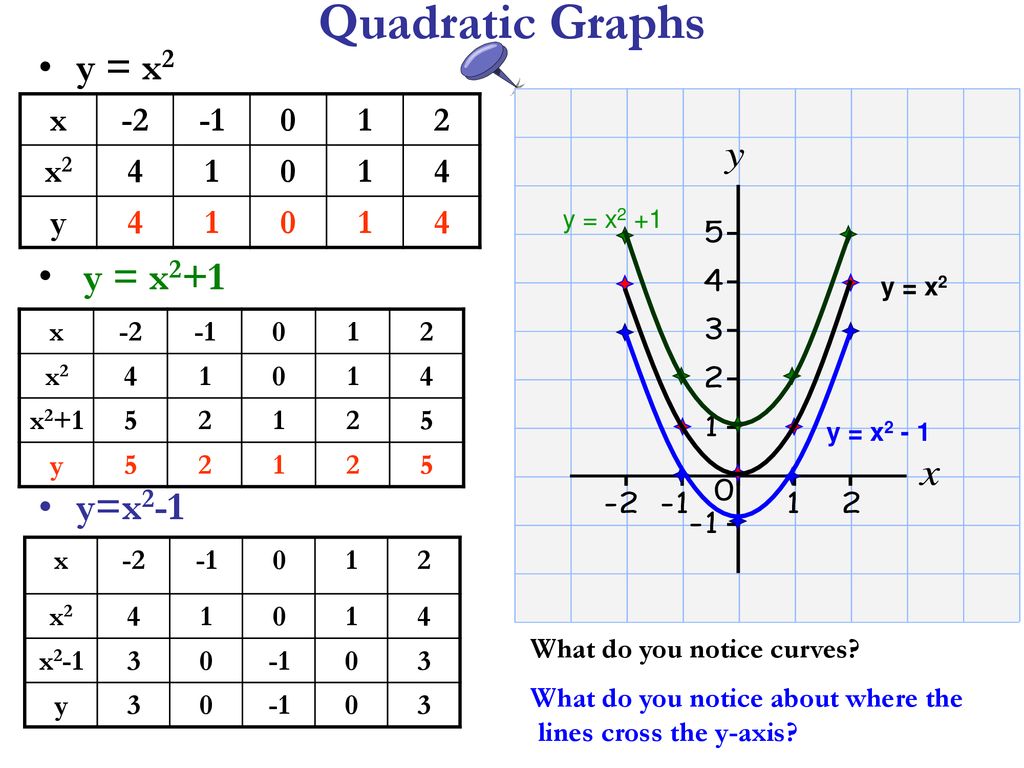
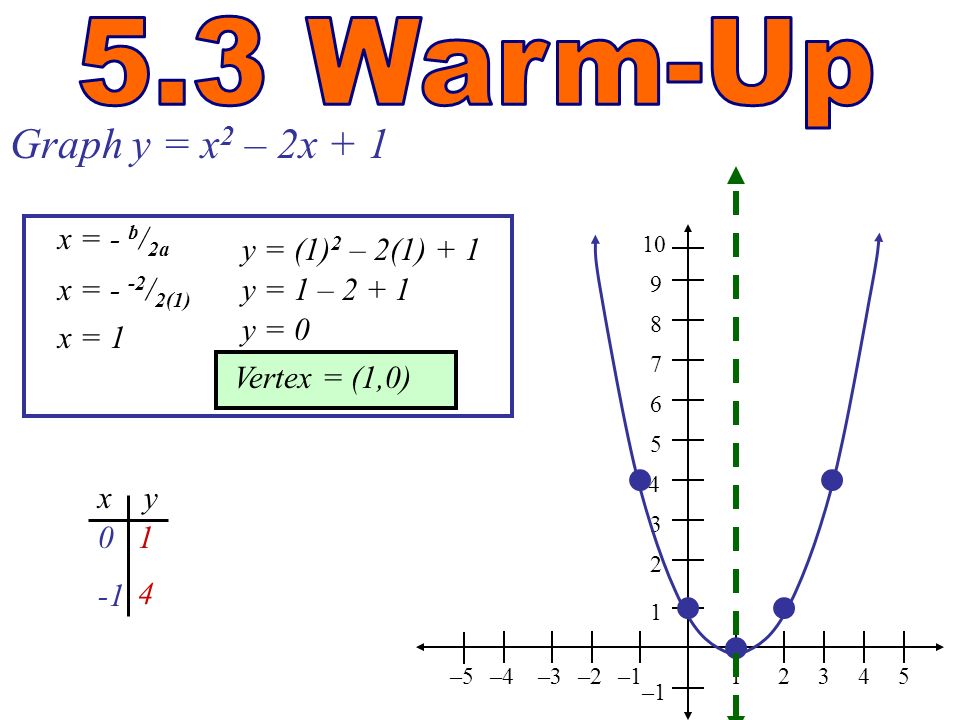
Graph (y2)^2=4(x1) Rewrite the equation as Divide each term by and simplify Tap for more steps Divide each term in by Cancel the common factor of The focus of a parabola can be found by adding to the xcoordinate if the parabola opensGraph the parabola, y =x^21 by finding the turning point and using a table to find values for x and yGraph the parabolas y = 1/2 (x1)^2 x=1/4 (y1)^2 2 y = 1/2 (x1)^2 This equation is a parabola of the form, y=A (xh)^2k For given equation, y = 1/2 (x1)^2
Graph y^2/4-x^2/9=1のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 |  | |
 |  | |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
 | ||
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
 | ||
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 | ||
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  |  |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 | 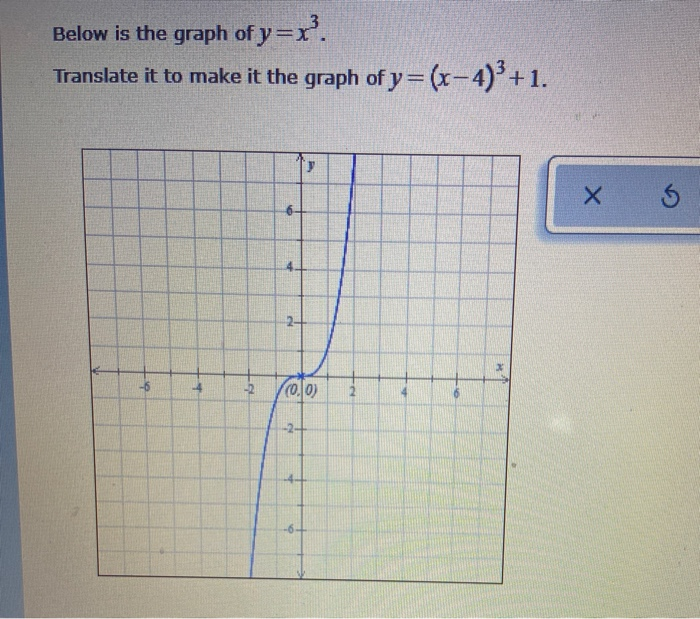 | |
 |  |  |
 |  |  |
「Graph y^2/4-x^2/9=1」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |
Explanation The general equation of a circle is (x −a)2 (y − b)2 = r2 The center is (a,b) and the radius is r (x −2)2 (y −1)2 = 22 This is a circle, center (2,1) and radius = 2 Mark the center at (2,1) then with a compass draw a circle of radius 2 graph { (x2)^2 (y1)^2=4 5847, 6637, 198, 426} Answer linkX^2y^2=1, (x2)^2(y1)^2=4 Natural Language;
Incoming Term: graph y-2=4(x+1), graph y^2/4-x^2/9=1, graph of y=x^2-4x+1,




0 件のコメント:
コメントを投稿